The mystery of the mutilated chessboard – Emma la pasticciera, a well-known lover of mathematical puzzles and problems of logic after a long study, decided to select the most beautiful logical-mathematical games of each time by proposing their solutions separately. These questions require both logical and creative skills to be solved. The perfect mathematical puzzle must propose a simple situation to explain and a solution just as easy to understand but very complicated to find, often at the limit of the impossible for an average mind.
In this page we present The mystery of the mutilated chessboard, another logic game (after The problem of the three switches) where you need to activate the c.d. lateral thinking… The term lateral thinking, coined by the Maltese psychologist Edward de Bono, means a way of solving logical problems that involves a particular approach, or the observation of the problem from different angles, as opposed to the traditional way of concentrating on a direct solution to the problem. A direct solution involves the use of sequential logic, solving the problem starting from the considerations that seem more obvious, lateral thinking departs from it (from which the lateral term) and seeks alternative points of view to seek the solution.
THE MYSTERY OF THE MUTILATED CHESSBOARD
Emma often remembers the first lesson in mathematics at the University where the professor, just entered the auditorium full of freshmen of the first year presented himself drawing on the board a grid-shaped checkerboard 8×8 and then two joint squares.
“This is an 8×8 board of 64 squares. This is a piece of domino whose dimensions are exactly those of two adjacent chessboard squares”.
“With 32 dominoes you can cover the board perfectly with 8 columns made of 4 dominoes placed vertically.”
“You don’t need a genius up to here!” the professor chuckled, wiping his chalk-stained hands.
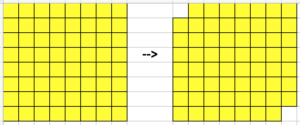
“The problem is that a friend of mine at that board sawed off two opposite corners – exclaimed the professor – so the mutilated board consists of 62 boxes”.
So the question is simple:
Is it possible to perfectly cover this chessboard with 31 pieces of the dominoes, without breaking them?
(The Solution is at the bottom of the page)
Musical interlude
Flying the Dolomites
Swings of Memory
Let’s Talk About Us
Solution
Come and discover the magical musical world of Lorenzo Pescini!
ART519 – All rights reserved- Pescini.com